Georgia Institute of Technology College of Sciences

The summer school is aimed at graduate students and recent Ph.D.'s. The goal is to introduce early-career researchers to the latest developments in the theory of hyperbolic polynomials, sums of squares and their applications in combinatorics and optimization. School of Mathematics Professor Greg Blekherman is the organizer. 

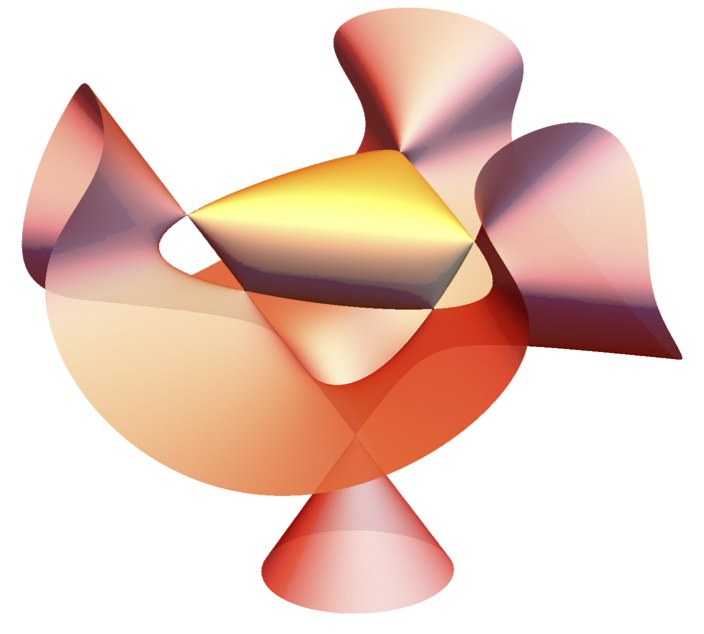
Hyperbolic and stable polynomials have seen several spectacular applications in combinatorics and optimization in recent years.

A hyperbolic polynomial in one variable is just a real polynomial with only real roots, while a hyperbolic polynomial in several variables can be seen as a familiy of such real-rooted polynomials in one variable. They appear in several different areas, and a beautiful geometric theory with many surprising features has evolved around their study. 

Nonnegative polynomials and sums of squares are classical subjects of real algebraic geometry, dating back to Hilbert's 17th problem. There are also rich connections to real analysis via duality and moment problems, as well as to polynomial and combinatorial optimization.

Topics

• Geometry of Hyperbolic Polynomials and Sums of Squares
• Conic and Hyperbolic Programming
• Interlacing Polynomials
• Stable Polynomials in Combinatorics
• Sums of Squares in Combinatorics and Optimization

Lecturers 

• Daniel Plaumann (TU Dortmund)
• Rainer Sinn (FU Berlin) 
• Cynthia Vinzant (NC State) 
• Greg Blekherman (Georgia Tech)

Funding: We have NSF funding for participants. Please visit Applications to apply.

Website: Check the website for updated information.